Electron Mass - Mass Of Electron, Charge, Speed, & Value Of Electron

Mass of Electron: Electron mass or electron rest mass is given. Approximately 9.10938356 × 10^-28 grams. and 9.10938356 × 10^-31 kilograms (kg). Mass of electron in gram, kilo gram, amu and electron Volt(eV) also given below

Contents

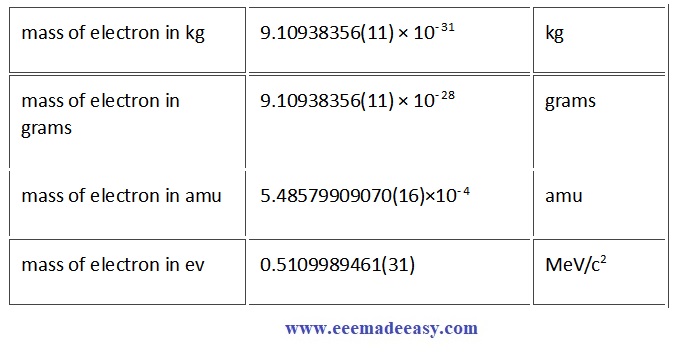
Rest Mass of an electron in grams,Kg,amu & EV table

mass of electron in kg	9.10938356(11) × 10⁻³¹	kg
mass of electron in grams	9.10938356(11) × 10⁻²⁸	grams
mass of electron in amu	5.48579909070(16)×10⁻⁴	amu
mass of electron in ev	0.5109989461(31)	MeV/c²

Electron, mass of electron, charge of electron, value of electron

The electron is a fundamental particle that plays a crucial role in the field of physics. It is a main building block of matter, and its properties have been extensively studied and scrutinized by scientists over the years. In this article, we will discuss about electrons, focusing on their mass, charge, speed and value.

Electron drift velocity

Basic Physical Concepts of Atom, Nucleus, Proton, electron is detailed in this post in EEE Notebook Read it HERE

I. The Electron

A. Definition of electron and Discovery:

The electron is a subatomic particle that carries a negative electric charge. It was first postulated by British physicist J.J. Thomson in the late 19th century. Thomson’s cathode ray tube experiments led to the identification of negatively charged particles, which he named electrons.

B. Role in Atomic Structure:

Electrons are fundamental components of atoms. They orbit around the atomic nucleus in specific energy levels, forming the basis of atomic structure. The arrangement of electrons determines the chemical and physical properties of elements and molecules.

II. Mass of the Electron मास ऑफ इलेक्ट्रॉन A Tiny But Significant Quantity

A. Experimental Determination:

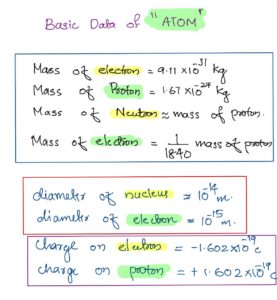
The mass of an electron is remarkably small, approximately 9.10938356 × 10^-31 kilograms (kg). The precise value has been determined through various experiments, such as the Millikan oil drop experiment and measurements using mass spectrometers.

B. Comparisons with Other Subatomic Particles

The electron is significantly lighter than other subatomic particles, such as protons and neutrons, which are found in the atomic nucleus. The mass of an electron is approximately 1/1836 times that of a proton.

III. Charge of the Electron: Nature’s Elementary Electric Charge

A. Millikan’s Oil Drop Experiment

The charge of an electron is -1.602176634 × 10^-19 coulombs (C), which is considered the elementary electric charge.

This value was determined by American physicist Robert A. Millikan through his famous oil drop experiment.

Millikan’s Oil Drop Experiment

Millikan's_setup_for_the_oil_drop_experiment

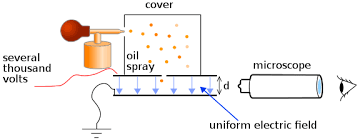
The oil drop experiment was performed by Robert A. Millikan and Harvey Fletcher in 1909 to measure the elementary electric charge (the charge of the electron).

The experiment took place in the Ryerson Physical Laboratory at the University of Chicago.Millikan received the Nobel Prize in Physics in 1923.

Millikan’s work provided the first accurate measurement of the electron’s charge.

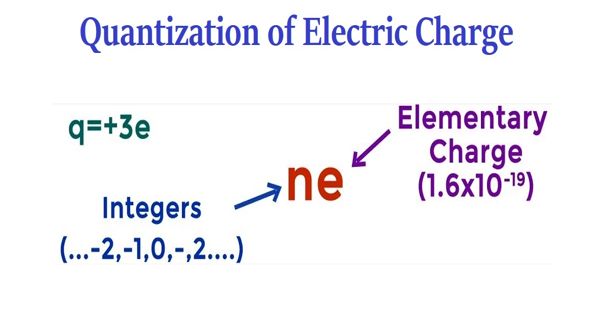
B. Quantization of Electric Charge

The charge of an electron is quantized.

All observable charges are always some integral multiple of elementary charge e = 1.6 × 10^-19 C . This is known as quantization of electric charge.

q = ne

Any accumulation or deficit of electric charge in a system is a result of the transfer or removal of electrons.

Quantization of Electric Charge eeemadeeasy

Unit of Charge- Coloumb

Coulomb is not a Practical Unit or it is Very Large Unit.
coulomb is a very large unit, hence practical units like milicoulomb (mC), microcoulomb(μC), nanocoulomb (nC) are used.

C. Significance in Electricity and Magnetism

The charge of an electron is central to the understanding and application of electricity and magnetism.

Electric current is the rate of change of electrons. The direction of current flow is opposite to the direction of flow of electrons.

IV. Value of the Electron: Implications in Modern Science

A. Electron Volt (eV)

The electron volt (eV) is a unit of energy commonly used in particle physics.

It is defined as the energy gained or lost by an electron when it is accelerated through an electric potential difference of one volt. One electron volt is equivalent to 1.602176634 × 10^-19 joules (J).

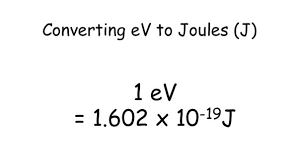
B. Relation to Avogadro’s Constant

The value of the electron’s charge plays a crucial role in determining Avogadro’s constant, which represents the number of entities (atoms, molecules, or particles) in one mole of a substance.

Avogadro’s constant is approximately 6.02214076 × 10^23 entities per mole, and it relies on the charge of a single electron.

C. Applications in Particle Physics

Understanding the properties of electrons, including their mass, charge, and value, is vital in the field of particle physics.

It enables scientists to develop advanced particle detectors, study particle interactions, and explore fundamental forces and the nature of matter.

Mass of electron in gram ,in kg, and give comparison with mass of proton and neutron

Mass of Electron

In grams: Approximately 9.10938356 × 10^-28 grams.
In kilograms: Approximately 9.10938356 × 10^-31 kilograms (kg).

Mass of Proton

In grams: Approximately 1.67262192 × 10^-24 grams.
In kilograms: Approximately 1.67262192 × 10^-27 kg.
Comparison: The mass of an electron is approximately 1/1836 times smaller than the mass of a proton.

Mass of Neutron

In grams: Approximately 1.674927498 × 10^-24 grams.
In kilograms: Approximately 1.674927498 × 10^-27 kg.

Comparison of mass of electron

The mass of an electron is approximately 1/1838 times smaller than the mass of a neutron.

It is important to note that while the masses of protons and neutrons are relatively similar, the mass of an electron is significantly smaller.

This disparity in mass plays a fundamental role in determining the overall characteristics and behavior of atoms and the interactions between subatomic particles.

Electron Weight

The weight of an electron can be calculated by multiplying its mass by the acceleration due to gravity.

However, it’s important to note that the weight of an electron is extremely small, given its minuscule mass. Here is the weight of an electron on Earth:

Weight of Electron on Earth

In newtons (N): Approximately 8.996464679 × 10^-38 newtons (rounded to the nearest decimal place).

Since the weight of an object depends on the gravitational force acting upon it, and the mass of an electron is incredibly small, the weight of an electron is essentially negligible in everyday scenarios.

Speed of Electron

it’s important to note that the concept of “speed” for electrons is somewhat different from macroscopic objects due to their wave-particle duality.

In a vacuum, electrons can reach speeds very close to the speed of light, which is approximately 299,792,458 meters per second (or about 186,282 miles per second).

Electrons can approach this speed in particle accelerators or in certain high-energy experiments.

Electrons typically drift at speeds on the order of millimeters per second in a typical electric current.

This drift velocity is much lower than their individual speeds due to frequent collisions with other electrons and atoms within the conductor.

So, the speed of an electron can vary depending on the context, from very close to the speed of light in certain high-energy scenarios to significantly slower speeds in everyday situations.

Electron drift refers to the net motion of electrons in a conductor under the influence of an electric field.

When a voltage is applied across a conductor, such as a wire, the electric field exerts a force on the free electrons within the material, causing them to move in a preferred direction.

Drift velocity

Drift velocity (v_d) is the average velocity of electrons as they drift in response to the electric field. It can be mathematically expressed using the following equation:

v_d = μ * E

where μ (mu) represents the electron mobility and E represents the applied electric field strength.

The value of the drift velocity depends on various factors, including the material properties and the strength of the applied electric field.

The electron mobility (μ) is a material-specific constant that quantifies how easily electrons can move in a given material.

It is typically measured in units of meters per second per volt per meter (m/s/V/m).

The drift velocity of electrons in most conductors is relatively low, on the order of millimeters per second.

For example, in copper, which is a commonly used conductor, the drift velocity at room temperature can be around 0.01 to 0.1 mm/s when subjected to a typical electric field strength of 1 volt per meter.

Electron-Mass of electron, Charge of electron, Speed of Electron, value of electron

It’s important to note that although the drift velocity of electrons is relatively low, the electric signal itself propagates at a much higher speed, close to the speed of light, due to the collective behavior of electrons and electromagnetic interactions within the conductor.

How to measure the speed of electron

Measuring the speed of an electron can be a complex task due to its small size, high velocities in some contexts, and the principles of quantum mechanics involved.

we can discuss a general approach that has been used in certain experimental setups:

Electron Beam:

One method to measure the speed of electrons is to create an electron beam and observe its behavior. This can be achieved by using a cathode ray tube or an electron gun. By controlling the electric and magnetic fields around the electron beam, its path and velocity can be manipulated.

Deflection in Electric and Magnetic Fields:

By subjecting the electron beam to known electric and magnetic fields, it is possible to measure the deflection or curvature of the beam.

By analyzing the extent of deflection and the known properties of the fields, the speed of the electrons can be calculated.

Time-of-Flight:

Another approach involves measuring the time it takes for electrons to travel a known distance. By knowing the distance and the time taken, the speed can be calculated.

This method is often used in particle accelerators, where electrons are accelerated to high energies, and their speed is determined by precisely measuring their time-of-flight.

Interference and Diffraction:

Interference and diffraction phenomena can also be utilized to indirectly infer the speed of electrons.

By observing the wave-like behavior of electrons, such as in electron diffraction experiments, the speed can be indirectly determined by analyzing the resulting patterns.

It’s important to note that the methods described above provide average speeds or statistical information about the speed distribution of electrons rather than individual electron speeds.

Additionally, the speed of electrons can be influenced by the specific conditions and the context in which they are measured, so the measured speeds can vary depending on the experimental setup.

Download & Install EEE Made Easy App